6 posts tagged with "thoughts"

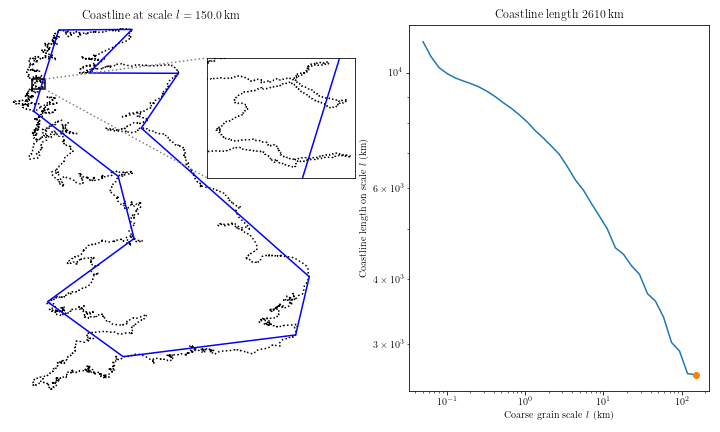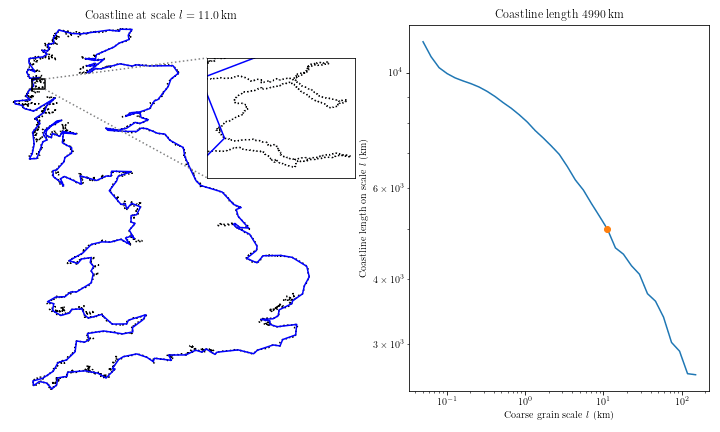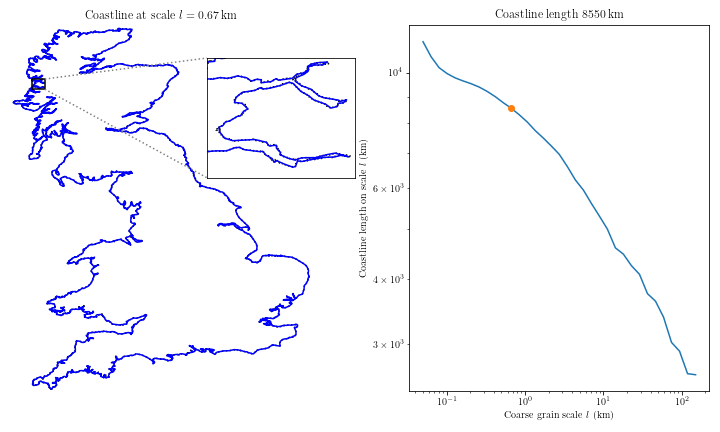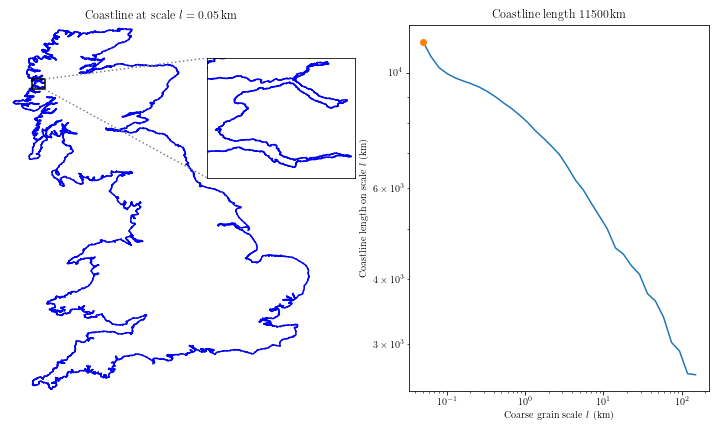
There is no shallow end to the philosophical pool.

-- P.F. Strawson

The correct view, I shall argue, is both the one left standing when we have seen how other views fail, and the one that answers best to these human concerns.

-- Derek Parfit, Vol II On What Matters

1.​

In this note I will briefly grapple with the question of what philosophy is and is not. As a part of answering this question, I will also engage with the question of why philosophical problems are so perennially perplexing. I include this because their perplexing aspect sheds light on what they are at heart. Both topics cover such a broad landscape that I can only hope to do a brief fly-over here.

2.​

Standard examples of philosophical questions are "What is truth?", "What is justice?", "Is there right and wrong in the world?", "Is everything a physical thing?" I will start by claiming that looking for some strictly defined common denominator to all of these questions is a mistake. There is unlikely to be any one ingredient the inclusion of which makes a question distinctly philosophical. It might be objected that they are not immediately answerable to experience and that this is the ingredient. While this is certainly in the mix, it won't do for distinguishing the philosophical from the non-philosophical unless we want to call all kinds of questions philosophical like "What would my life have been like if I grew up in France?", "When is the best time to have children?", or "Was this actually the best move, or did I just get lucky?". While we can think of superficially experience-related answers to these questions, the chain of reasons we would justify our answers with terminates in reasons that involve values or abstract commitments. Conversely, some branches of philosophy are almost entirely comprised of confrontations with immediate experience, phenomenology, for example.

3.​

There are two claims we could make about a supposed essence of philosophical discourse.

• That some discourse includes $X$ , automatically makes it philosophical. (has $X$ $\rightarrow$ is philosophical)
• Having $X$, is a necessary condition for some discourse to be philosophical. (is philosophical $\rightarrow$ has $X$ )

The first seems fruitless, beyond the boundaries drawn by historical contingencies or university departments. The second it seems, can only be given a fuzzy definition:

• is philosophical $\rightarrow$ probably has $X$

There are some ingredients that seem to give us evidence that some discourse is philosophical, but it seems mostly connotational. "That question is one that philosophers tend to ask." is about all we can say on the matter with a high degree of confidence.

4.​

This brings me to the claim I will defend in this note about the "essence" of philosophical discourse. I claim that there is no type of discursive move one can make that is distinctly philosophical and no type of discursive move one can make that is distinctly not philosophical. That is to say, philosophical discourse is a member of a fuzzy set, or has a family resemblance with, all other types of cognitive (truth-apt) human discourse. Science, common sense, mathematics, philosophy, explaining the rules of a game, describing how to be a better actor, choosing which lure might catch more fish this afternoon, etc are all different human discursive acts that draw pluralistically from various toolkits in order to get some job done.

5.​

I am not denying that each practice listed above has probabilistically distinctive ingredients. I am denying that we have necessary and sufficient conditions for when one or the other is being done. The overwhelming majority of science involves empirical testing, but so does trying out a new recipe in the kitchen or the philosophical thought that it seems like we have free will. Science implicitly makes philosophical commitments and engages in conceptual engineering. Philosophy, when it does consult experience, frequently draws on the most basic and immediate experience, not "experiments" as we might think of them in science.

6.​

Where do we get the idea that it seems like we have free will? From merely understanding a concept?

7.​

If all discourse is "philosophical" (in some weak sense), because philosophical discourse shares more ingredients with other types of discourse than it has distinctly, then is all discourse "mathematical" and "scientific" as well? I think the names of these different realms of discourse do not denote something distinct that we are doing when we do them as much as they signify that types of discourse or thought belong to certain contexts. I am claiming that these different realms share much more in common than they do not.

8.​

That physical systems are causally closed, that all facts are physical facts, that every event has an explanation, that parsimonious explanations are to be preferred over less parsimonious ones, that only empirically verifiable results are to be expected from respectable science, and that the true demarcation of science from non-science is falsifiability. All of these claims, should we accept any of them, are not themselves empirical claims.

9.​

From a history of philosophy perspective, it is interesting that all attempts to do away with philosophy by some sweeping (usually philosophical) thesis end up with intractable problems of self-reference. The verificationist criteria is not itself verifiable. The entire Tractatus "cannot be said". Naturalists who reject metaphysics in place of physics must rely on non-naturalist principles to do so. And, more recently, radical pragmatist/post-modernist efforts to show that all claim to truth is merely a product of culture must rely on statements that seem to transcend culture to make that move (if it is to avoid being self-defeating).

10.​

If methods define the discipline, then we aren't going to get much clarity. Philosophy uses "scientific methods", and science uses "philosophical methods." Of course, I am not saying that there should be papers in Nature about "Synthetic A Priori" truths or papers in Mind about microbiological experiments. We have good connotational definitions, and I don't think we need better ones than the ones we have and employ.

11.​

Maybe we want to say that the philosopher when she makes deflationary a claim about a particular philosophical problem because it has gone unsolved for hundreds of years, is temporarily doing history? Is the philosopher qua historian actually doing empirical science because they are using the 'inductive method'? After all, such arguments are called 'pessimistic inductions'. Maybe the economist who implicitly excludes the possibility of strongly emergent non-physical events being the cause of some market trend is doing a bit of philosophy?

12.​

I see philosophy as just mapping out the inferential relationships between concepts we commit ourselves to by virtue of understanding/mastering those concepts. Or, making new/revised concepts and exploring the relationships those new concepts bear to ones already in use. Why should that be simple? Why should even the blueprint for how to do that be simple?

13.​

In any claim that we make, we are broadcasting to others what commitments we are making and what inferences can/should be drawn from what we say. This is, of course, a non-distinctive attribute of philosophical discourse.

14.​

When philosophy (or any other discipline in pursuit of truth) engineers concepts, I think the goal should be understanding. Understanding of what is the case, what would be the case if $P...$, what is necessarily the case, what is possibly the case, what is probably the case, and so on for all of the modalities (or perhaps, as many philosophers have thought, only some of the modalities, the others being reducible to those).

15.​

I think Strawson is correct in the opening quote to this note. So one might wonder: if Strawson is right and the philosophical pool has no shallow end, and there is nothing distinctive about the methods with which philosophy explores the implication-space of concepts we currently have (or create/modify), then why do these questions have no "shallow end"?

16.​

It is uncontroversial to say, and a testable empirical claim, that philosophical problems have evaded solution and dissolution by humans for thousands of years. Why? To continue roping some current reading material in here, I will bring in several of Eric Schwitzgebel's potential answers to that question from The Weirdness of the World (he frames the issue specifically in the context of metaphysics):

Possibilities as to why metaphysical problems seem to resist easier answers:

$1$. "Without some bizarreness, a metaphysics just wouldn't sell."

$2$. "Metaphysics is difficult." (There is a metaphysics out there with no Bizarre implications)

$3$. "Common sense is incoherent in matters of metaphysics."

$4$. (Adding one which Schwitzgebel brushes aside) "Metaphysical problems are pseudo-problems that are not truth-apt."

I was once convinced by $4$, but after feeling tensions in those ideas to be unresolvable, I now believe that some combination of $2$ and $3$ is the case. More precisely, I believe $2$ is true and $3$ is true, $3$ compounding the difficulty in $2$.

12.​

If this account is correct so far, then the discipline concerned with the connotationally philosophical problems is just one instance of the bringing-under-the-microscope of various conceptual claims and commitments that we make (implicitly or explicitly in our words and practices). Since those practices are complex and multifarious, it isn't unreasonable to expect the resulting web of commitments and entailments we get from exploring or engineering concepts in that web to be equally complex.

13.​

Why are philosophical problems hard? Philosophical claims (connotationally demarcated as the questions philosophers tend to ask) involve complex constellations of commitments between concepts that share a high amount of inferential connections with others but are weakly determined by our least rejectable workaday beliefs.

14.​

There is no shallow end of the pool because commitments about concepts (like knowledge or mind) have broad-reaching implications on nearly everything else we believe, but at the same time, particular commitments about those concepts are not strongly forced on us by experience or immediate necessity. Those broad-reaching implications are likely to collide with some other commitments in the common-sense picture of the world and these collisions form the many points of perennial philosophical debate. They are often collisions with our least rejectable beliefs, such as "I have free will". At the same time, since our experience can be made to fit many different philosophical views by shifting other commitments, we are rarely forced to choose one conclusion. Rather, we can spend a lot of time exploring all the various collisions with common-sense, or with other commitments we have, to find the most desirable set of concepts, commitments, and entailments. This, I believe, is simply a massive and combinatorially tremendous problem to solve.

15.​

Which theory of truth you endorse is unlikely to change your views about whether it is Wednesday or whether the moon landing was faked. It might change how you view the world quite significantly, though, over time. Total conceptual inefficacy does not follow from workaday inefficacy. There is some best theory to complement our understanding, but it will play out in a vast network of other commitments.

16.​

The route to understanding is like deciding the right path through something like the following decision tree:

• $G$ is something like the conceptual scheme, categories of experience, and resulting commitments that we have forced upon us by experience and our constitutions as human beings.
• Branches directly from $G$ are the various conceptual commitments we could reasonably take from that "starting point".
• Downstream (outward) nodes are the commitments entailed by those choices.
• Downstream (outward) branches are the subsequent conceptual decisions we can make as a result of those upstream commitments.
• Every decision path is reversible (we disavow certain commitments and undertake others)
• Taking one path is committing to a claim ("All things are physical things") or to a rule that defines a concept (e.g. to "be a pentagon is to be a plane figure with five straight sides and five angles" or to "having free will is to be an unmoved mover")
• We can turn back on commitments, rationally, for many reasons:
  • Recalcitrant experience
  • Conceptual inconsistency
  • Contradiction
  • Parsimony, to name only a few...

Ideal understanding would be achieved in this mock world when all commitments that $G$ is taken to entail are handled in such a way that makes the least collisions, internal contradictions, and inconsistencies. This may include any subset of the various possible branches that can carry us to some Peircian ideal. In the final understanding, we are left with the commitments that are, as Parfit says, the ones "left standing when we have seen how other views fail, and the ones that answer best to these human concerns" and which are, in the Peircian sense, subjunctively to be agreed upon by robust inquiry.

We must "test" each route to the periphery from $G$ by exploring the implications of each conceptual decision and seeing whether it collides with any upstream commitments or is rejectable for any of the above reasons. In the connotationally philosophical problems, few of the major choices are impacted by experience forcibly, and there is little cost to reversing our choices. We can expect this endeavor to understand to converge on truth only very slowly.

This may seem like a foundationalist picture, but it is not intended to be. $G$ is merely something less assailable (maybe by many magnitudes) than outer nodes and should probably be represented by a cluster of nodes. In reality, things are far more complex and the web of commitments is far larger and more labyrinthian. It is likely something more like a fractal version of this diagram where each node has in itself a vast depth of self-similar structure.

17.​

This picture has problems, clearly. I would not fully endorse it as an adequate picture of understanding. It is only meant to capture the nature of inquiry based on how claims entail other claims and how a structure of beliefs that is revisable, has much more up for revision the farther we get from $G$-like beliefs.

18.​

Those familiar with Quine's Web of Belief, might find the seeming inversion of the structure here confusing. Quine placed the more "revisable and "closer" to experience beliefs at the web's periphery. My little picture here is not a "web" but rather a node network of decision trees. I am representing something like the following. One could imagine each arm extending from $G$ as representing a realm of understanding. If it were the branch concerning metaethics, it might look like this:

And so one can imagine a branch for ethics, physics, aesthetics, philosophy of mind, cosmology, mathematics, and so on.

19.​

Under this view, expecting to solve major philosophical problems with ease would be as unlikely as solving any other combinatorial explosive problem to complete satisfaction. Consider just how combinatorially explosive things can become. Are there more moves in the real game of inquiry, than in the game of chess (which has a game-tree complexity of about $10^{120}$)? The problem is worse for several reasons:

• In chess, our making or not making certain moves does not entail much about any other moment of our lives or the lives of others. In the game of inquiry, our moves very much do.
• Individual moves/decisions in the game of inquiry are much less certain. How sure are we really that Non-Cognitivism is true? Might we not have to, over the course of many thinker's lives, work out lots of that decision tree's branches to be sure which account is the best "one left standing when we have seen how other views fail, and that answers best to human concerns"?
• In our incredibly simplified mock game of inquiry, there are no cross-discipline commitments. Nothing in the "epistemology" tree can collide or enforce anything in the "metaphysics", "metaethics", or "physics" tree. Surely this is not the case in human thought. Realistically, it probably looks like a much more chaotic version of this:

20.​

Consider what it is to "bite the bullet" in this mock game of inquiry. We take an upstream commitment that seems dubious to avoid the net more dubious downstream commitments of another branch.

21.​

The above is a faint sketch which could doubtless be filled in with much greater detail. For now I think it suffices to capture how I view philosophy, in a broad sense. I believe this sketch has strong merits. First, it allows us to explain why philosophical problems are truth apt without invoking empirically dubious differences between the different truth-apt human discursive practices and without appeals to weird mental faculties of platonic intuition. Second, it shows us why philosophical problems are expected to be very complex to solve. They are conceptually far-reaching, yet weakly determined by small portions of the decision tree in the game of inquiry. So, most of the time, experimentation cannot be expected to close the case.

22.​

These difficulties, however, do not make the solutions to philosophical problems any less truth-apt. To throw in the towel early is a sign of hubris. It is to mistake complexity for an illusion or a impassible barrier. Claims are best deemed not to be truth-apt, non-cognitive, when there is no conceivable grounds for their being considered true or false. This may apply to some philosophical problems. But conceivability is not possibility and, as I have argued, conceptual revision does take place. Even for the most intractable nonsense-seeming philosophical problems we have ever heard, we cannot responsibly say that no good sense could ever be given to the words. Obviously, though, there are better and worst problems to spend one's time on.

Philosophy begins in wonder. And at the end when philosophic thought has done its best the wonder remains.

-- Alfred North Whitehead

In philosophy, if you think the answer is obvious, you haven't understood the question.

-- Keith Frankish

1.​

I have decided to experiment with a new format here. It often takes me longer than I'd like to write/edit essay-style writing on this site. My goal in building and maintaining this little thing was always to solidify philosophical ideas of mine that were sort of just floating around in my thinking or scribbled in a notebook somewhere.

2.​

The new format is this. Numbered chunks of a few sentences or paragraphs. Just having numbered "thoughts" or "propositions" or whatever you want to call them is certainly a less organized mode of capturing thought, but I think it also wins over the essay in several important categories. I've borrowed this style from several philosophical heroes of mine and I think it served them well and maybe even enhanced my understanding of their ideas.

3.​

One change that has come over me philosophically in the last several years is an emphasis on not expressing/having complete certainty on most philosophical issues and being ok with that. I think that intellectual humility in the face of issues that have puzzled humans for thousands of years is the correct response. Empirically speaking, we should take seriously the notion that philosophical problems have been around for as long as humans have and have resisted simple conclusions. For me, this change amounts to a shift from "I think X" to "I assign a high credence to X".

4.​

In my personal experience, the less someone acknowledges tensions between their various philosophical commitments and the more convinced they are that have it all figured out, the less powerful their problem-solving seems to be. (I include parts of myself and previous iterations of myself in there.)

5.​

I used to fear thinking about philosophical problems and forbid myself from thinking about them because I knew certainty was unlikely to be found. The floor would fall out from under me. This has always discomforted me, and sometimes, I would be up until 2 in the morning, puzzling over something. Rather than fearing to play the game just because an all-out win was unlikely, I have come to enjoy the playing of the game.

6.​

I have recently been reading Eric Schwitzgebel's The Weirdness of the World. This book has significantly reinforced the themes of epistemic humility and withholding some certainty in philosophical thinking for me. In this book, Schwitzgebel claims that the proposed solutions to most major philosophical problems have Universal Bizzarness and Universal Dubiety. The former, by Schwitzgebel's definition, means that these proposed solutions have implications contrary to common sense and the latter means that these proposed solutions do not resoundingly compel belief. I think he is correct. Why that is the case is an interesting question for later, perhaps.

7.​

This new format accomplishes two things, one reflecting my changing philosophical attitude and one practical. First, it reflects some of the uncertainty involved in tackling philosophical problems in its denial of opening-body-conclusion type of thinking. Second, it makes creating these notes less time-consuming and lowers the pressure a bit, which I hope can speed up the process and make it more fun.

8.​

I hope to be every bit as precise in my thinking with this new format, but perhaps to relax a bit more about essay structure and completeness of "answers".

Alice: "I don't know what you mean by 'glory.'"

Humpty Dumpty: "Of course you don't -- till I tell you. I meant 'there's a nice knock-down argument for you!'"

Alice: "But 'glory' doesn't mean 'a nice knock-down argument.'"

Humpty Dumpty: "When I use a word, it means just what I choose it to mean -- neither more nor less."

Alice: "The question is whether you can make words mean so many different things."

-- Lewis Carol, Alice in Wonderland

Introduction: What is Ordinary Language Philosophy​

I take Ordinary Language Philosophy ($OLP$) to be any philosophical program that claims that the problems of philosophy are best solved (or, in some cases, completely solved) by paying attention to ordinary language. As the Wittgenstein of Philosophical Investigations says:

Philosophical problems arise when language goes on holiday.

(Though Wittgenstein spawned an era of $OLP$, it is arguable whether he himself was a through-and-through $OLP$ philosopher.)

An example of tackling a philosophical problem using $OLP$ might be as follows. We might wonder "What is triangularity?" Sure we see triangles, but we don't "See triangularity". In comes the $OLP$ philosopher to insist that looking for a "thing that we see" in place of triangularity is a mistake. We should, they will say, pay attention to how we use the word in ordinary language, and we will see that we have made what Gilbert Ryle would call a category error in assuming that our singular term "triangularity" should refer to something like "That balloon" does. They will say that we can avoid being so misled by sticking to our ordinary language guns and not letting language "Go on holiday".

In this short note, I hope to outline where I think $OLP$ goes wrong and why, and also what it gets right. I will argue that $OLP$ is correct in the following points:

and I will argue that $OLP$ is incorrect and should be polished up in the following points:

I think $OLP$ is an immensely powerful tool and like all powerful tools, it is prone to overuse and misapplication. Although I once believed it, I have long since abandoned the idea that all philosophical problems can be resolved by appealing to ordinary language.

What Does Ordinary Language Philosophy Get Right?​

You can't get semantics before pragmatics. Meaning is use.​

We cannot pick the meanings of our words out of thin air. So much the worse for Carol's Humpty Dumpty. They depend on learning sets of concepts. It often seems like philosophers are doing this when particular philosophical puzzles arise. An example that tends to bother me is when philosophers fall prey to the notion that we can sort of "mean things at will". They do so when they say, for example, "Maybe the red I see is what you see when I see blue!" How exactly do we mean "red" in that sentence? In the same way that we learn how to use the word "red"! And, if that is the case, then it seems like I cannot be meaning it "in some other way at the same time" that presumably only I could understand. Or if I am, then that way of meaning that just sits flaccidly alongside the pragmatically efficacious one (the one that has cash-value of meaning in our actions) and isn't really a part of communicating with others.

It is as if when I uttered the word I cast a sidelong glance at the private sensation, as it were in order to say to myself: I know all right what I mean by it.

-- L. Wittgenstein, Philosophical Investigations $\S$ 274

I don't have preconceptual, in this case prelinguistic, knowledge of what "red" is and so how could I possibly mean something by it that is directly out of accord with the pragmatics of that concept (How I learned to apply it). I might as well say, "maybe the feeling I get when I take a bath is the same as the one you get when you have a toothache".

$OLP$ is correct to steer us away from these illusory meanings and the knotty philosophical problems that come with them. I think it is correct, not by virtue of the primacy of ordinary language, but by focusing on what it is that gives our expressions meaning in the first place.

Theoretical meanings have to be related somehow to pragmatic meanings or they are useless.​

When we are giving some kind of theory, predictive, descriptive, synoptic, or prescriptive, the theory will have no use to anyone if it is not tied to a concept that has pragmatic value to us. I think the free will issue is illustrative of this kind of divorce of theoretical meaning from pragmatic meaning. My typical argument with the determinist who acts like they are the first person to discover that the physical world of science is causally closed goes something like:

It should seem peculiar to us that "real choices", on the determinist's view, turn out to be just the sort we don't have. The pragmatics of what is and isn't a "choice" have gone entirely out the window and with them the notion of what we are talking about having any material consequence. And so the conversation will circle back to "What is a choice?" and the determinist and compatibilist will disagree on that. But why should we care about this particular determinist's definition of choice? It seems like they have a big "So what?" to answer for next if we accept it. When we divorce theoretical "choice" from what practices and actions we take in knowing and deploying the word "choice" in real life, what importance does the theory really have?

$OLP$ is correct to keep us on a slightly tighter leash than we sometimes want to be on when philosophizing. We need to stay somewhat tethered to the common-sense concepts if we want our philosophizing to pertain to them.

If meaning comes from use, then meanings will be an imprecise hodge-podge because "doing" and therefore "use" is an imprecise hodge-podge. This is why "conceptual analysis" has never successfully analyzed a concept in the history of philosophy.​

We should assign an extremely low probability to the possibility of 'fully analyzing a concept'. In an effort to make the concept precise and immune to counterexamples, we will confine the concept to a tiny playpen and thereby commit the mistake of divorcing the concept from the vast landscape of actions and practices that give it meaning at all. We either have a chaotic hodge-podge of a concept, a fragile one to counter-examples, or one so surgically defined that it simply has nothing to do with the concept we sought to elucidate in the first place. I believe accepting the hodge-podge is the best play here.

That doesn't mean we can never have precision in more tightly bounded domains. It only means that precision across the board in every practice and context, as the determinist above thinks that can have with "choice", will likely be impossible and should not be expected. If meaning is determined by what we do, and what we do changes, how can we expect to ever catch up?

If meanings come from uses, then they must follow rules. For communication to be possible, some normativity must be assumed.​

As Wittgenstein (under some interpretations) and Kripke point out, in their rule-following paradoxes, applying a concept properly, learning a word, following a rule cannot be something that is done all by oneself in a vacuum. Kripke asks us to imagine a rule for a mathematical function called quus. Quus, $⊕$, is just like 'plus', $+$, except:

$x⊕y = x+y$

if $x, y < 57$

but

$x⊕y = 5$

if $x, y > 57$

Suppose I have been teaching you to add all the way up until we have gotten to:

$57 + 57$

What should you answer? $5$? or $114$? The point of the strange example is that it seems like nothing about how I have taught you to add so far has determined whether I meant quus or plus. There is no fact of the matter. The same skeptical challenge could be applied to any rule and so it seems that without some turning of the spade and reaching justification for the rule, this could go on ad infinitum. We reach an Agrippan trilemma of "rule following". It seems no rule can ever be justified merely by example or stipulation of how the rule should be followed because any action can be made to fit the rule and any rule made to fit my past actions. If we need rules for how to follow the rules, then how could a rule ever ground a practice? Wouldn't we then need "rules for following rules for following rules"?

I think the rule-following paradox terminates in doing. We don't learn rules by painstakingly memorizing instructions or "rules for following rules".

Someone says to me: "Show the children a game." I teach them gambling with dice, and the other says "I didn't mean that sort of game." Must the exclusion of the game with dice have come before his mind when he gave me the order?

-- L. Wittgenstein, Philosophical Investigations $\S$ 70

In this silly example, when the concerned parent says "Not that kind of game!" he didn't consult an inner rule or mental lookup-table of any kind in order to exclude gambling from the kind of games he meant. The meaning of the sentence and the rule that we were to follow was grounded in practice, in the pragmatic "doing of stuff", in habits and the innate nature of us as human beings.

Ordinary language is rough around the edges, does a million more things than state facts about the world, constantly changes, and is a reflection of what we do and what we fundamentally believe must be done. There is no playing the game of philosophy without language or concepts of some kind which set their roots in practice. Therefore, we have to philosophize while keeping in mind that those words and concepts are constructions of the practices and actions that give them meaning in the first place.

What Does Ordinary Language Philosophy Get Wrong?​

Ordinary use has no theoretical primacy. A term can grow to encompass more than its ordinary use.​

I am often frustrated reading $OLP$ philosophers say things like "This is a misuse of the word thought". I am all for taking the semantics as following the pragmatics. We cannot just mean whatever we want by words without getting the ball rolling through some kind of action in practice. If I want "thought" to mean "words I speak internally", then I am certainly allowed to adopt that as a technical term. It may not relate very well to the ordinary use of the word or concept, but maybe that isn't my goal in whatever particular mode of philosophizing I am doing.

$OLP$ philosophers have attacked neuroscientists' use of sentences like "The brain thinks X". They claim this is a misuse of "to think" as only persons can think. That's where the pragmatics come from they claim, and I agree. We learn to say "think" of someone based on how they are acting etc etc. However, I think it is silly to claim that this kind of metaphorical talk is "nonsense" full stop. We very frequently use intentional language as a proxy to quickly understand complex systems and that should stand on its own theoretical merit, not be judged by the stipulated authority of ordinary language.

What claims ordinary language sentences should/shouldn't,do/don't commit us to are not known implicitly. They must be accounted for theoretically.​

There seems to be a sentiment in $OLP$ that all philosophy should do is describe how terms are used. However, in reading some of these philosophers, Ryle particularly, I often noticed that they seemed to be doing a lot more than that. More specifically, I often notice that they argue for or take for granted certain theories of how we should account for a given way of speaking.

These supposed "mere descriptions" from $OLP$ philosophers are often just expedient theories of how something in the world works. Sometimes they are empirical and need evidence, sometimes they are empirical and don't need evidence (because they are so obvious), and sometimes they are synoptic/prescriptive accounts of how language should be viewed to work. Here's an example: Quine (another philosopher who puts pragmatics before semantics) gives the example of "sakes" as a word that takes the grammatical form of a singular term but does not refer to anything. There are no "sakes" to be accounted for. Did we automatically know that there weren't "sakes" by virtue of knowing how to say "for Pete's sake"? Is that question "nonsense"? Is Quine giving a theory of how our words should be viewed? I think he is and so is every $OLP$ philosopher who prompts us to focus on the ordinary use of a word to circumnavigate a philosophical problem. The theory is still a theory whether we got it from observations about ordinary language or from philosophical accounts after the fact. We cannot know all there is to know about the application of a concept merely by looking at its current use because that concept's application is a prescriptive, not a purely descriptive endeavor. Should we say "it is the same time here as on the moon" in a post-relativistic physics world? Ordinary use cannot give us the answer. In a sense, our concepts are in a constantly changing state. We are almost always engaging in some small amount of conceptual engineering every time we encounter a novel case. But don't get me wrong. I'm all in favor of eschewing "accounts of" something when the ordinary concepts will do just fine.

We don't know all the implications of our claims just by virtue of making or being disposed to make them. Additionally, we don't always know how the ordinary concepts we use hang together, or how they relate to one another. We can be masters of the concepts of "inference" and "belief" without ever having thought about how they relate.

The pragmatic move is a good move, but it does not instantaneously give us a correct and satisfactory account of how our terms work, how their use can be more technically refined, or how they do/should relate to the word as science tells us it exists.

Not all philosophical problems are dissolved in ordinary language as it evolves and keeps up with science.​

Even in $OLP$ with a strong adherence to not extending ordinary terms beyond their common use, it isn't clear to me why all philosophizing is off limits by virtue of it not having some property that ordinary language has. I'd agree with Wittgenstein that lots of philosophical problems arise from our "bewitchment by language" (non-referring singular terms being a good example), but it is far from clear to me that we can say all of them are.

Philosopher David Enoch has a very fun example to illustrate this. In Pulp Fiction, Vincent Vega and Jules Winnfield are having a little back-and-forth about why Jules doesn't eat pork.

So by that rationale, if a pig had a better personality, he would cease to be a filthy animal. Is that true?

Enoch points out that both speakers are clearly philosophizing. It seems wrong to me to say that they just crossed the precipice of sense and were talking nonsense to each other even though they thought they weren't. On the other hand, I do think it is possible to philosophize in vain by playing games with words that are too far gone from their practical application for the inferences we might validly make about them to have any relevance at all. I take philosophizing to be a general extension of the basic human discursive practice of making our commitments to certain claims clear, whether to ourselves or to one another. That's what Jules is doing, and that is what I am doing now.

A fractal is a way of seeing infinity

-- Benoît Mandelbrot

Nothing is built on stone; All is built on sand, but we must build as if the sand were stone.

-- Jorge Luis Borges

Can You Measure a Coastline?​

In 2006, the Congressional Research Service (CRS) of the United States estimated the coast of Maryland to be 31 miles long. Strangely, the National Oceanic and Atmospheric Administration (NOAA) estimated it to be 3,190 miles long. This staggering difference was not a clerical error. These values were the actual reported measurements from each study of the Maryland coastline. (For more see here)

Maryland's is not the first coast in history that cartographers have separately measured to wildly different lengths. The phenomenon is known as the "Coastline Paradox". When estimating the length of any fractal curve via straight lines, the actual length is indeterminate. Suppose I measured the coastline of Maryland with a yardstick, and you with a foot-long ruler. My answer will be far smaller than yours. Without getting into the math, the smaller your measurement-length, when measuring a fractal curve, the larger the length of that curve will be.

This measurement problem is known as the Richardson Effect and has been studied in detail by Benoît Mandelbrot. Mandelbrot famously described shapes with infinitely embedded and repeating complexity with very simple equations that describe what he called "fractals".

I will not go through the exact definition of a fractal here, but we can simply say for now that a fractal curve is defined as one whose perceived complexity changes with measurement scale.

In Mandelbrot's own words a fractal is, loosely:

a rough or fragmented geometric shape that can be split into parts, each of which is (at least approximately) a reduced-size copy of the whole

When measuring any fractal shape, real or abstract, we face this coastline problem. Part of what it is to measure is to agree on something. It is on the basis of this embedded self-similarity of fractals that the "Coastline Paradox" is claimed to be a "Paradox". It is claimed that a coastline has no real determinate length, yet we can measure it to have some length.

Is there really a paradox here? I think in a trivial sense yes. I argue that real objects that exhibit fractal curves only have a determinate length insofar as we can agree on a measurement schema. Do we stop at the grain of sand? The Angstrom? The inch? The best answer will be determined by our goals, but that does not mean it will always be easily attained. It has been pointed out recently, on account of this goal-based agreement, that perhaps a coastline is not really fractal in the Mandelbrot sense.

A Strange Philosophical Question​

My purpose in this note is not mathematical or cartographic. I want to consider the "Coastline Paradox" as a model for our understanding of reality and pose some philosophical questions based on that comparison.

Mandelbrot considered his fractals to be related to a "theory of roughness". The relationship between a fractal edge and our concept of 'roughness' is easy to see in a physical object. Imagine zooming in on an aerial view of a coastline. The closer we get, the more the shape changes, and embedded patterns are revealed that are self-similar to the overall shape (in some sense, perhaps not exactly as in Koch Snowflake GIF at the start of this note).

The philosophical question I want to ask is this: what if our relationship to reality is analogous? What if the deeper we dig into some phenomena or some realms of understanding, the more embedded complexities we discover ad infinitum? This question decomposes into the questions of whether there is some infinite nature to the depth of inquiry and whether that infinite depth would exhibit some self-similarity the deeper it went. I will focus mostly on the question of the potentially infinite depth of inquiry, where depth refers to gaining more knowledge that is not merely about the knowledge that one already has.

I will argue that the answer to the question: "could inquiry could be infinite?" highlights the goal-dependent nature of inquiry and understanding generally and that if there is a Richardson Effect to finding more facts, then goal-dependent and more pragmatist frameworks for metaphysics and epistemology are better ones. Just as the real Coastline "Paradox" resolves when we agree on a measurement criterion, so the philosophical skepticism of how we could know anything or be acquainted with any reality truly if it is in some deep sense fractal can be sidestepped when we share goals in understanding the world.

Clarification​

Admittedly, the general philosophical question of this note is, so far, unclear.

What if the relationship of inquiry to reality is fractal, like the relationship of a measured length to a coastline?

Let's clarify it a bit. There are two ways to interpret this question.

• Metaphysical Fractality

  Reality IS such that infinitely more detailed descriptions of phenomena will always be true of it.

This would mean that, whether we can know it or not, there is no rock-bottom to inquiry. The more facts we would discover, whether we were to discover them or not, the more other facts there will be to discover. It is important to note that, in order for this thesis to be interesting at all, these additional facts would not merely be "facts about the facts we already have", that is trivially true. The more facts we get, the more facts about facts there are. Fractal 'roughness' is not merely recursion.

Perhaps an example will help this point. Suppose physicists discover $G$-ons (some particle that describes all the other higher-level phenomena we know about) and the behavior of all the other things in the universe now makes sense. But wait! If Metaphysical Fractality is true, then we will likely subsequently discover that $G$-ons are composed of $H$-ons and $I$-ons etc etc and the process of discovery only moves 'downward' infinitely.

• Epistemological Fractality

  Our knowledge of reality is (and will be) such that a more detailed account will always avail itself (eventually) whenever a new less detailed one does.

Epistemological Fractality would mean that there is something, perhaps something necessary, about the nature of our knowledge and its relation to the world that makes it never reach able to reach rock-bottom, but always peels back another layer revealing new knowledge infinitely. Again, to be clear, it is trivially true the more knowledge we get, the more knowledge about that knowledge there is. That is not what is meant by Epistemological Fractality.

With either Epistemological Fractality or Metaphysical Fractality being true, a "coastline problem" emerges for our knowledge of the world. If Metaphysical Fractality is true, no matter what the nature of our inquiry is, there will never be an absolute fact that is not an infinite composite of deeper facts. If Epistemological Fractality is true, something about knowledge, but maybe not reality, yields the same effect.

Setting our metaphysical and epistemological baggage down for a minute, these two theories will be identical in most if not all of their predictions. Also, Depending on one's account of truth, these two theories might sound identical altogether. To avoid confusion and stick to the point here I will therefore treat them as the same.

I will cut a lot of philosophical corners here and revise our question to this:

Is either reality itself, or any possible knowledge we could have about it, fractal in nature? Is inquiry of infinite depth?

Perhaps to answer in the affirmative is just to adopt a certain disposition towards inquiry.

How Can We Answer?​

How might we answer the above question? Perhaps empirically? We can rephrase our question above as an empirical hypothesis. But is it actually testable? Its infinite nature muddles the meaning of its testability. Alternatively, maybe a rule that we firmly believe in guides us the conclusion that reality is fractal?

Empirically?​

Suppose, to continue with our mock-physics example, that we continue on to discover $Z$-ons, $A_2$-ons, $B_2$-ons, and eventually to $Z_2$-ons... $A...Z_{1001}$-ons.

The evidence always confers the same probability, at some observation $n$, on "infinite observations" as it does on "$n$ observations". So is it impossible to empirically decide? In the case of adding more observations, yes. However, in the case of observations completely ceasing it seems not. We could imagine a case where we discovered $Z_{1001}$-ons and that just tied everything up. That's it. Understanding is complete at the discovery of the $Z_{1001}$-on. Surely some will find this view absurd, but it is certainly possible unless we take a rather hardline commitment to Epistemological Fractality (or Metaphysical Fractality where we are ignorant of reality for some compelling reason).

If we admit that such a hard stop is possible and if we never observe such a hard stop, which we have not yet, then we have increasing evidence for reality being fractal with each layer of reality we peel back (but we do not have more evidence for it being fractal than for it only going as far as our present depth). This may or may not be significantly dependent on what prior probability we assign to reality being fractal in this way.

A Rule?​

The Fibonacci sequence is infinite. How do I know that? I know it because the Fibonacci numbers are the sequence of numbers

$\{F_n\}_{n=1}^\infty$

where

$F_n = F_{n-1} + F_{n-2}$

with $F_1 = F_2 = 1$, and conventionally defining $F_0 = 0$.

I know the rule to get the next number. Take the last two numbers, add them together and I get the next.

Given $0, 1, 1, 2$, I add $1+2$ and get $3$. It seems that I know that this can never end because I know a rule that, by virtue of my following it, necessarily gives me a new Fibonacci number.

Do we know such a rule for inquiries about reality generally: that they will always give me more facts or more questions left open? I do not think so (again, not in the sense of more 'facts about the facts', for that is trivially true). It seems necessarily true that the Fibonacci sequence will never terminate if I sat down and cranked out numbers for an infinite amount of time. It does not, in any salient way, seem necessarily true that one discovery unearths at least one more. However, if reality's fractality is not necessarily true or false, it follows as a simple truth of modal logic that it is possibly true.

An Answer​

Whether some rule would emerge that would conclusively show us that reality is or is not fractal or whether some hard-stop/rock-bottom would be observed that would prove that it is not, at present it seems that we have to accept that it is possible that reality is fractal in the sense suggested above.

The cash-value of believing that reality is possibly fractal with respect to inquiry, is a stance of preparedness for endless complexity and a disregard for the importance of ultimate ontological questions. For, if it is true that reality is possible fractal, then what there is, in reality, has no determinate answer unless we agree on a criterion, as in the coastline problem, for what we care about.

I think this highlights an already common-sense view. Who cares what the 'ultimate truth' of the coastline's length is if we are just trying to sail a boat around the coast of England in some finite amount of time? Who cares what the 'ultimate constituents of reality' are if we are just trying to survive, do good, launch a rocket, cure diseases, do the laundry, help future humans survive and flourish and so on. Only within these inquiries would it be worthwhile and possible to have an agreed on criterion of measurement (supposing reality is of this infinite fractal nature). Once we have such a criterion of measurement we no longer face indeterminacy about the answers.

This subject has in no way been treated with exhaustive clarity here and much more could be said about it. For the time being, I find it a fascinating lens for contemplating our relation to a world that is possibly intractably complex as creatures of finite attentive and computational power.

Death is nothing to us, since when we are, death has not come, and when death has come, we are not.

-- Epicurus

Perhaps the greatest contradiction of our lives, the hardest to handle, is the knowledge "There was a time when I was not alive, and there will come a time when I am not alive."

-- Douglas Hofstadter, Gödel, Escher, Bach

Sadness about what happens after one dies makes sense when it is about the experiences of others, as in 'How will my grandchildren feel when I am dead?' Does it make sense in reference to one's self?

There are enigmatic assertions and feelings regarding death that initially seem to be about one's own experience yet evidently cannot be upon further inspection. Clearly, 'How will I feel once I have died?' is a nonsensical question. It has always somewhat confused me that people express sadness toward the fact that at some point they 'will have died' when that sadness is supposed to be on their own behalf.

Most adult humans have had a thought like 'At some point, I will have died, and isn't that sad!' This thought is only able to make us sad because it presents us with a convincing illusion. We imagine ourselves standing beyond our own death, still somehow having experiences, and thinking back with a feeling of loss and nostalgia at our own lives.

This feeling of loss, I will argue, evaporates under closer inspection. The following argument shows that sadness about one's own death on behalf of one's self is irrational or actually about others. I hope it lifts in the reader the convincing illusion that compels us to feel a false sense of loss on behalf of our future selves.

When you are dead, I believe, everything that could meaningfully be called 'you' is gone. Until some such possibility as uploading one's consciousness to a computer or molecule-for-molecule clones, the existence of the self beyond death is only for the realm of thought experiments and science-fiction.

This conclusion should not be interpreted as bleak, however. Instead, it should focus our attention to the things that do actually matter after we die, which will be for those then living to experience. I am not a solipsist and I think some things matter even when we are gone. We should not think about the illusion of how our 'dead selves' will feel, but instead we should think about how we will leave the world for those who are still there when we are gone.

It has always seemed odd to me to hear people say "Wow. That was 10 years ago." or "Time flies so fast." While I empathize with the feeling, my inner response is usually something like "Every second of your life took place across one second, no?" So why does it feel like time moves so fast in retrospect?

The reason is that human memory uses a form of data compression. There is even some evidence to suggest that consciousness itself is an efficient compression method. All memories are partial, they are made up of fewer bits than their initial cognitive representations needed. If that were not the case, all memories of an experience would be virtually identical to having that experience again.

Here's a quick example

Say we have this data:

In run-length encoding, consecutive repeated characters are replaced with the character itself followed by the count of its repetitions.

Assuming we are using ASCII encoding, where each character is represented by 8 bits (1 byte):

To be clear, I am not merely making the obvious claim that "time passes at a constant rate". Instead, I am saying that due to the way our memory compresses information, the more time we are alive the more 'compressed memory' we will have and the 'faster' it will appear that time flies by. Our actual present experience is always full and our recollection of past experiences always partial. This creates the illusion that we should fear our actual present experiences 'slipping away faster and faster'.

It always helps to assuage the negative feelings I get when contemplating that "time moves quickly" to realize this simple truth. It helps me be present. That time moves any quicker than it actually takes place, as one accrues memories, is an illusion. Maybe that thought can help others too.